

In incompressible fluid dynamics, dynamic pressure is the quantity defined by:
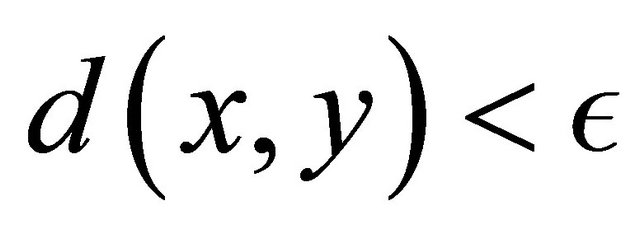
In the high velocity flow through the constriction, kinetic energy (dynamic pressure – ½.ρ.v 2) must increase at the expense of pressure energy (static pressure – p).Īs can be seen, dynamic pressure is dynamic pressure is one of the terms of Bernoulli’s equation. This lowering of pressure in a constriction of a flow path may seem counterintuitive, but seems less so when you consider pressure to be energy density. This effect causes the lowering of fluid pressure (static pressure) in regions where the flow velocity is increased. The term dynamic pressure (sometimes called velocity pressure) is associated with fluid flow and with the Bernoulli’s effect, which is described by the Bernoulli’s equation: In general, pressure is a measure of the force exerted per unit area on the boundaries of a substance. Total and dynamic pressure are not pressures in the usual sense – they cannot be measured using an aneroid, Bourdon tube or mercury column. Static pressure + dynamic pressure = total pressure (stagnation pressure) The simplified form of Bernoulli’s equation can be summarized in the following memorable word equation: In the high velocity flow through the constriction, kinetic energy (dynamic pressure – ½.ρ.v 2) must increase at the expense of pressure energy ( static pressure – p).
The Bernoulli’s effect causes the lowering of fluid pressure (static pressure – p) in regions where the flow velocity is increased. Static pressure is one of the terms of Bernoulli’s equation: The term static pressure is identical to the term pressure, and can be identified for every point in a fluid flow field. In fluid dynamics, many authors use the term static pressure in preference to just pressure to avoid ambiguity. The dimensions of terms in the equation are kinetic energy per unit volume. In the high velocity flow through the constriction, kinetic energy must increase at the expense of pressure energy. This effect causes the lowering of fluid pressure in regions where the flow velocity is increased. The Bernoulli’s equation describes the qualitative behavior flowing fluid that is usually labeled with the term Bernoulli’s effect. This equation is the most famous equation in fluid dynamics. Under these conditions, the general energy equation is simplified to: the equation relates the states at two points along a single streamline (not conditions on two different streamlines).no change occurs in the internal energy,.no heat is transferred to or from the fluid,.density is constant (which also means the fluid is incompressible),.Bernoulli’s equation has some restrictions in its applicability, they summarized in following points: It puts into a relation pressure and velocity in an inviscid incompressible flow. It is one of the most important/useful equations in fluid mechanics. The Bernoulli’s equation can be considered to be a statement of the conservation of energy principle appropriate for flowing fluids.


 0 kommentar(er)
0 kommentar(er)
